CAPACITORS AND ALTERNATING CURRENT
The four parts of figure 4-3 show the variation of the alternating voltage and current in a capacitive circuit, for each quarter of one cycle. The solid line represents the voltage across the capacitor, and the dotted line represents the current. The line running through the center is the zero, or reference point, for both the voltage and the current. The bottom line marks off the time of the cycle in terms of electrical degrees. Assume that the ac voltage has been acting on the capacitor for some time before the time represented by the starting point of the sine wave in the figure.
Figure 4-3. - Phase relationship of voltage and current in a capacitive circuit.
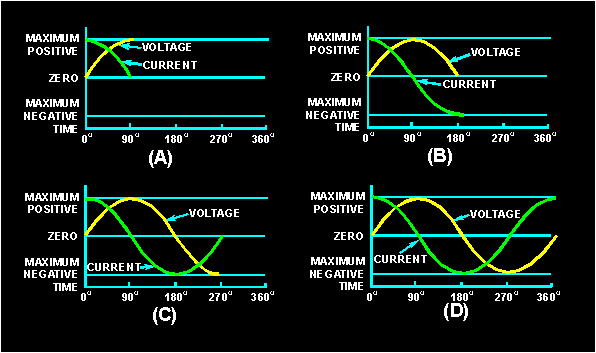At the beginning of the first quarter-cycle (0° to 90°) the voltage has just passed through zero and is increasing in the positive direction. Since the zero point is the steepest part of the sine wave, the voltage is changing at its greatest rate. The charge on a capacitor varies directly with the voltage, and therefore the charge on the capacitor is also changing at its greatest rate at the beginning of the first quarter-cycle. In other words, the greatest number of electrons are moving off one plate and onto the other plate. Thus the capacitor current is at its maximum value, as part (A) of the figure shows.
As the voltage proceeds toward maximum at 90 degrees, its rate of change becomes less and less, hence the current must decrease toward zero. At 90 degrees the voltage across the capacitor is maximum, the capacitor is fully charged, and there is no further movement of electrons from plate to plate. That is why the current at 90 degrees is zero.
At the end of this first quarter-cycle the alternating voltage stops increasing in the positive direction and starts to decrease. It is still a positive voltage, but to the capacitor the decrease in voltage means that the plate which has just accumulated an excess of electrons must lose some electrons. The current flow, therefore, must reverse its direction. Part (B) of the figure shows the current curve to be below the zero line (negative current direction) during the second quarter-cycle (90° to 180°).
At 180 degrees the voltage has dropped to zero. This means that for a brief instant the electrons are equally distributed between the two plates; the current is maximum because the rate of change of voltage is maximum. Just after 180 degrees the voltage has reversed polarity and starts building up its maximum negative peak which is reached at the end of the third quarter-cycle (180° to 270°). During this third quarter-cycle the rate of voltage change gradually decreases as the charge builds to a maximum at 270 degrees. At this point the capacitor is fully charged and it carries the full impressed voltage. Because the capacitor is fully charged there is no further exchange of electrons; therefore, the current flow is zero at this point. The conditions are exactly the same as at the end of the first quarter-cycle (90°) but the polarity is reversed.
Just after 270 degrees the impressed voltage once again starts to decrease, and the capacitor must lose electrons from the negative plate. It must discharge, starting at a minimum rate of flow and rising to a maximum. This discharging action continues through the last quarter-cycle (270° to 360°) until the impressed-voltage has reached zero. At 360 degrees you are back at the beginning of the entire cycle, and everything starts over again.
If you examine the complete voltage and current curves in part D, you will see that the current always arrives at a certain point in the cycle 90 degrees ahead of the voltage, because of the charging and discharging action. You know that this time and place relationship between the current and voltage is called the phase relationship. The voltage-current phase relationship in a capacitive circuit is exactly opposite to that in an inductive circuit. The current of a capacitor leads the voltage across the capacitor by 90 degrees.
You realize that the current and voltage are both going through their individual cycles at the same time during the period the ac voltage is impressed. The current does not go through part of its cycle (charging or discharging), stop, and wait for the voltage to catch up. The amplitude and polarity of the voltage and the amplitude and direction of the current are continually changing. Their positions with respect to each other and to the zero line at any electrical instant-any degree between zero and 360 degrees-can be seen by reading upwards from the time-degree line. The current swing from the positive peak at zero degrees to the negative peak at 180 degrees is NOT a measure of the number of electrons, or the charge on the plates. It is a picture of the direction and strength of the current in relation to the polarity and strength of the voltage appearing across the plates.
At times it is convenient to use the word "ICE" to recall to mind the phase relationship of the current and voltage in capacitive circuits. I is the symbol for current, and in the word ICE it leads, or comes before, the symbol for voltage, E. C, of course, stands for capacitor. This memory aid is similar to the "ELI" used to remember the current and voltage relationship in an inductor. The phrase "ELI the ICE man" is helpful in remembering the phase relationship in both the inductor and capacitor.
Since the plates of the capacitor are changing polarity at the same rate as the ac voltage, the capacitor seems to pass an alternating current. Actually, the electrons do not pass through the dielectric, but their rushing back and forth from plate to plate causes a current flow in the circuit. It is convenient, however, to say that the alternating current flows "through" the capacitor. You know this is not true, but the expression avoids a lot of trouble when speaking of current flow in a circuit containing a capacitor. By the same short cut, you may say that the capacitor does not pass a direct current (if both plates are connected to a dc source, current will flow only long enough to charge the capacitor). With a capacitor type of hookup in a circuit containing both ac and dc, only the ac will be "passed" on to another circuit.
You have now learned two things to remember about a capacitor: A capacitor will appear to conduct an alternating current and a capacitor will not conduct a direct current.
The four parts of figure 4-3 show the variation of the alternating voltage and current in a capacitive circuit, for each quarter of one cycle. The solid line represents the voltage across the capacitor, and the dotted line represents the current. The line running through the center is the zero, or reference point, for both the voltage and the current. The bottom line marks off the time of the cycle in terms of electrical degrees. Assume that the ac voltage has been acting on the capacitor for some time before the time represented by the starting point of the sine wave in the figure.
Figure 4-3. - Phase relationship of voltage and current in a capacitive circuit.
As the voltage proceeds toward maximum at 90 degrees, its rate of change becomes less and less, hence the current must decrease toward zero. At 90 degrees the voltage across the capacitor is maximum, the capacitor is fully charged, and there is no further movement of electrons from plate to plate. That is why the current at 90 degrees is zero.
At the end of this first quarter-cycle the alternating voltage stops increasing in the positive direction and starts to decrease. It is still a positive voltage, but to the capacitor the decrease in voltage means that the plate which has just accumulated an excess of electrons must lose some electrons. The current flow, therefore, must reverse its direction. Part (B) of the figure shows the current curve to be below the zero line (negative current direction) during the second quarter-cycle (90° to 180°).
At 180 degrees the voltage has dropped to zero. This means that for a brief instant the electrons are equally distributed between the two plates; the current is maximum because the rate of change of voltage is maximum. Just after 180 degrees the voltage has reversed polarity and starts building up its maximum negative peak which is reached at the end of the third quarter-cycle (180° to 270°). During this third quarter-cycle the rate of voltage change gradually decreases as the charge builds to a maximum at 270 degrees. At this point the capacitor is fully charged and it carries the full impressed voltage. Because the capacitor is fully charged there is no further exchange of electrons; therefore, the current flow is zero at this point. The conditions are exactly the same as at the end of the first quarter-cycle (90°) but the polarity is reversed.
Just after 270 degrees the impressed voltage once again starts to decrease, and the capacitor must lose electrons from the negative plate. It must discharge, starting at a minimum rate of flow and rising to a maximum. This discharging action continues through the last quarter-cycle (270° to 360°) until the impressed-voltage has reached zero. At 360 degrees you are back at the beginning of the entire cycle, and everything starts over again.
If you examine the complete voltage and current curves in part D, you will see that the current always arrives at a certain point in the cycle 90 degrees ahead of the voltage, because of the charging and discharging action. You know that this time and place relationship between the current and voltage is called the phase relationship. The voltage-current phase relationship in a capacitive circuit is exactly opposite to that in an inductive circuit. The current of a capacitor leads the voltage across the capacitor by 90 degrees.
You realize that the current and voltage are both going through their individual cycles at the same time during the period the ac voltage is impressed. The current does not go through part of its cycle (charging or discharging), stop, and wait for the voltage to catch up. The amplitude and polarity of the voltage and the amplitude and direction of the current are continually changing. Their positions with respect to each other and to the zero line at any electrical instant-any degree between zero and 360 degrees-can be seen by reading upwards from the time-degree line. The current swing from the positive peak at zero degrees to the negative peak at 180 degrees is NOT a measure of the number of electrons, or the charge on the plates. It is a picture of the direction and strength of the current in relation to the polarity and strength of the voltage appearing across the plates.
At times it is convenient to use the word "ICE" to recall to mind the phase relationship of the current and voltage in capacitive circuits. I is the symbol for current, and in the word ICE it leads, or comes before, the symbol for voltage, E. C, of course, stands for capacitor. This memory aid is similar to the "ELI" used to remember the current and voltage relationship in an inductor. The phrase "ELI the ICE man" is helpful in remembering the phase relationship in both the inductor and capacitor.
Since the plates of the capacitor are changing polarity at the same rate as the ac voltage, the capacitor seems to pass an alternating current. Actually, the electrons do not pass through the dielectric, but their rushing back and forth from plate to plate causes a current flow in the circuit. It is convenient, however, to say that the alternating current flows "through" the capacitor. You know this is not true, but the expression avoids a lot of trouble when speaking of current flow in a circuit containing a capacitor. By the same short cut, you may say that the capacitor does not pass a direct current (if both plates are connected to a dc source, current will flow only long enough to charge the capacitor). With a capacitor type of hookup in a circuit containing both ac and dc, only the ac will be "passed" on to another circuit.
You have now learned two things to remember about a capacitor: A capacitor will appear to conduct an alternating current and a capacitor will not conduct a direct current.
No comments:
Post a Comment